If you have a load with a variable or poorly specified resistance and want to regulate the power applied to it (a heater for example), merely controlling the voltage or current will not work, as in both cases the power P = I2R = V2/R depends on R.
Instead, let us generate pulses with constant energy EPULSE, independent of the resistance of the load RL. Then by changing the frequency f of the pulses we can conveniently and precisely control the load power (P = f·EPULSE), from 0 to a known maximum level.
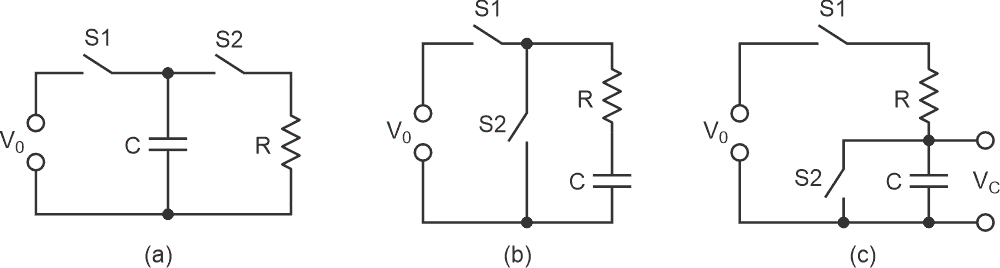
Figure 1a shows a simple way to generate a constant energy pulse. Capacitor C is charged to an initial voltage V0, storing ½CV02 joules. It is then discharged through the load. The pulse has a constant energy that does not depend on RL.
 |
|
| Figure 1. | Constant energy impulses. |
Such a simple approach has drawbacks. First, it is wasteful. In order to charge the capacitor with ½CV02 joules, another ½CV02 joules are lost (see Appendix for details).
The circuit of Figure 1b fixes that problem – all resistive losses now occur in the load.
One thing to note is that the power distribution of the pulse is quite uneven. About 63.2% of the energy is delivered in the first ½τ (τ = RL·C; energy lost in the load evolves twice as fast as the voltage rises, hence the factor ½). It takes a further ½·4τ for the next 36.1% of energy, which is equivalent to only about 1/7 of the average power during the first ½τ. The uneven power distribution limits the maximum power that can be controlled by the circuit. It takes infinity to transfer the remaining 0.67% of the energy. In practice this will be ignored, limiting accuracy.
However, as shown in Figure 1c, we can interrupt charging after the capacitor has reached a certain threshold value, VC. The energy dissipated in the load is equal to CVC·V0 – ½CVC2 and is again independent of the value of RL (see Appendix for derivation).
When choosing the value of VC, the main consideration is overshoot, which causes the energy of the pulse to be higher than calculated. The slew rate at the moment the threshold is reached is equal to:
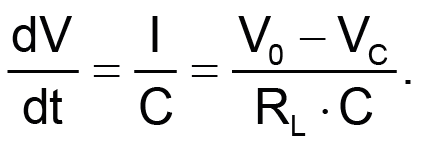
A lower threshold value VC results in higher overshoot for the same comparator speed.
The Design Idea in Figure 2 shows one possible implementation. A 555 provides switches, a comparator, and logic. The trigger input is biased above the trip point by divider R1 & R2. Triggering pulses pass through a small capacitor, C1 (2-10 pF), in order to prevent saturation of the comparator (see 8.3.1 of the datasheet). The maximum operating frequency is therefore comparable to that of an oscillator.
 |
|
| Figure 2. | Basic implementation of the constant power circuit. |
The R3-R4 divider sets the threshold voltage. A TL431 shunt regulator (see datasheet for the selection of R6-R8) sets the charge voltage. Depending on the chosen values, R5 (a couple of ohms) may be needed to prevent excessive current in the shunt regulator. The actual charge voltage will be higher by the forward voltage drop of D1. Use a fast diode for D1 and a film capacitor for C.
With a more elaborate control circuit, the wasteful discharge to ground can be eliminated. For example, capacitor C can be made to charge from –V/2 to +V/2 through RL from a positive rail V0 – V/2 and discharge through RL to a negative rail –V0 + V/2, creating a symmetrical pulse for both charge and discharge, and no wasted energy. Alternatively, the voltage of the capacitor can be made to oscillate around a midpoint above ground.
This design was used to construct a power supply for a thermally driven MEMS.
Appendix
Figure 3 shows a circuit where a capacitor is charged from a potential V0 to a potential VC. Charges moving from a higher to a lower potential perform work W = q∆V.
Charge is discrete, so we can look at the contribution of each individual charge. Such a charge starts from a state of rest at potential V0 at the source and ends up at rest on the plate of the capacitor at a certain lower potential VC.
 |
|
| Figure 3. | Charging a capacitor. |
If capacitor C is completely discharged initially, the work done by each charge follows the pattern:
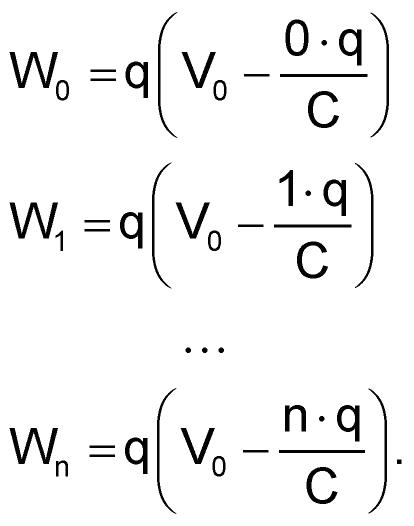
Here, n·q is the total charge Q on the capacitor after n individual charges, and
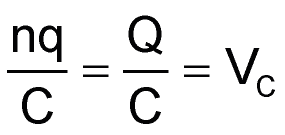
is the voltage to which the capacitor is charged. From that relationship we determine the number of charges:
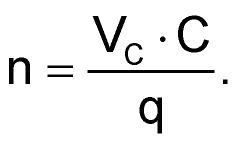
The total work is equal to the sum of the contributions of each charge: W = W0 + W1 +…+ Wn. Expressed as a function of VC, the work W(VC) is then equal to:
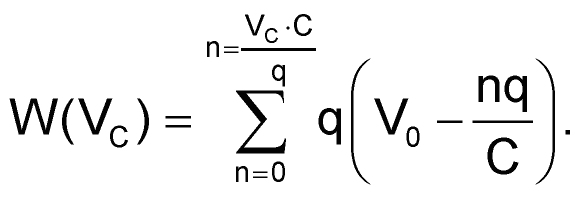
Simplifying, we obtain:
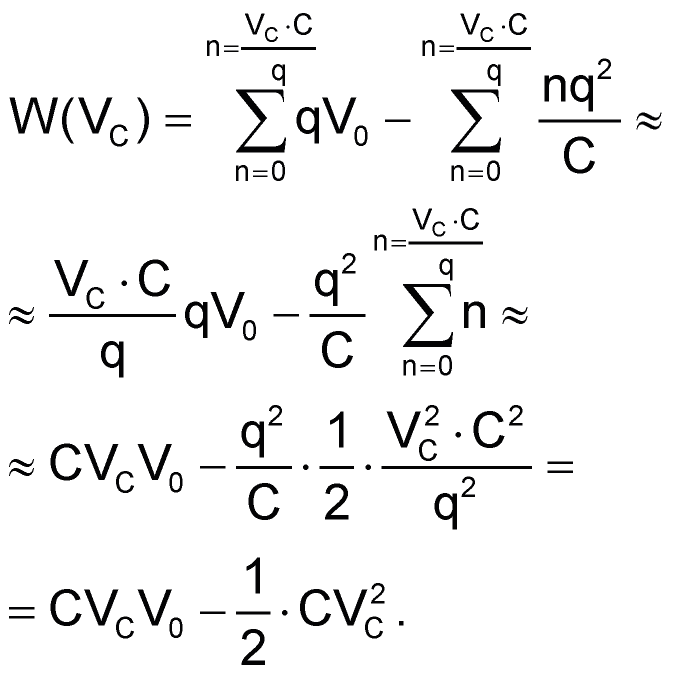
The first part stands for the energy taken from the voltage source. The second part is the energy which the capacitor holds when charged to a voltage VC. The difference is the energy lost, converted to heat in the load. As the equation shows, it does not depend on R in any way.
If we charge the capacitor to the full potential of the source, VC equals V0. The source then supplies CV02 joules, the capacitor holds ½CV02 joules, and another ½CV02 joules are converted to heat.