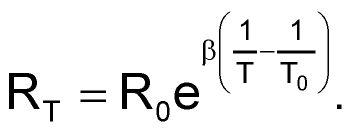Given their generally low cost, small size, robust construction, accuracy, versatility, and sensitivity, it’s no wonder that basic negative temperature coefficient (NTC) thermistors rate among the most popular temperature sensors available. However, their temperature response function is highly nonlinear (literally exponential), making excitation and signal digitization and processing interesting design exercises.
 |
|
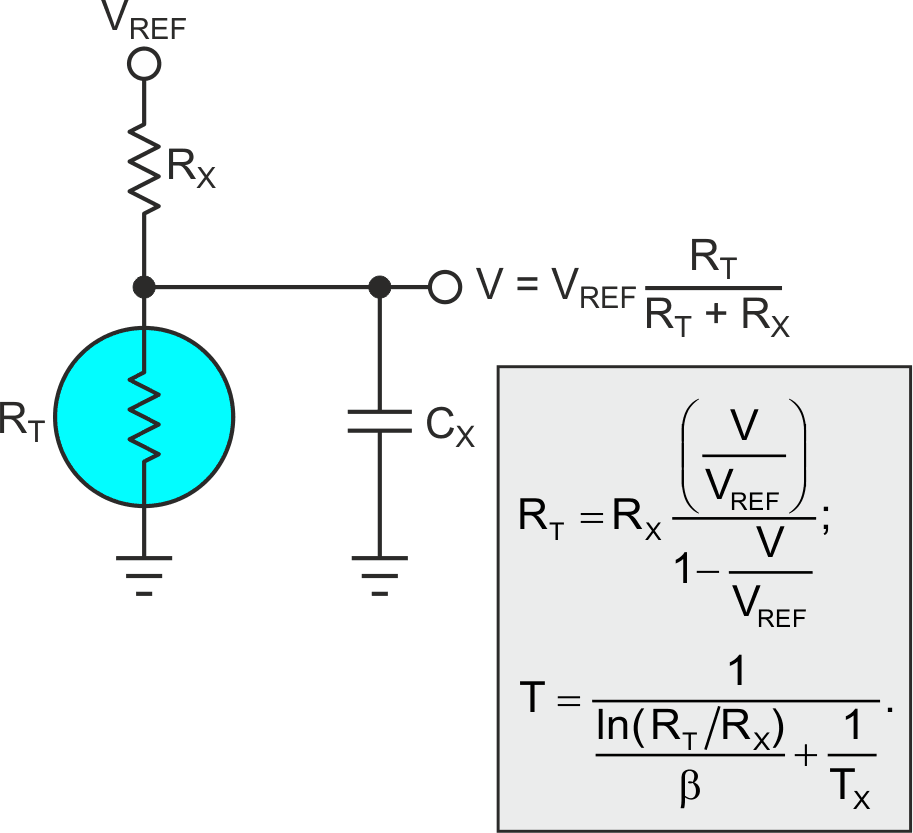
| Figure 1. | Basic thermistor passive excitation circuit: CX = optional noise reduction, perhaps 100 nF; RX = excitation resistor. |
The typical NTC thermistor’s datasheet (e.g., Molex 2152723605) summarizes thermo-electric properties with four parameters (Equations 1 through 5), shown in Figure 1 (numbers borrowed from 2152723605 data):
- T0 = rated/calibration temperature (25 °C = 298.15 K)
- R0 = resistance at T0 (10k ±1%)
- β = beta (3892 K)
- Dissipation (self-heating) factor (1.5 mW/°C)
Then thermistor resistance (RT) as a function of temperature (T) in Kelvin is predicted by:
Applying the classic KISS principle, we see in Figure 1 a candidate for the simplest possible circuit to wheedle a signal from a thermistor, and some basic math to winnow a temperature measurement from its output and parameters 1, 2, and 3 from above.
Other than the (very uncritical) CX and the thermistor itself, the only component in Figure 1 is RX. How best to choose its value?
Intuition suggests and math confirms that the optimum (at least nearly so) choice is to make RX equal to the thermistor’s at the middle of the span of temperature measurement required by the application. Said mid-point temperature (call it TX) will then output V = VREF/2 and thus distribute ADC resolution symmetrically over the range of measurement. Equation 5 tells us how to get there.
Suppose we choose a measurement range of 0 °C to 100 °C, then TX = 50 °C = 323.15 K and Equation 5’s arithmetic tells us (using the 2152723605’s numbers):
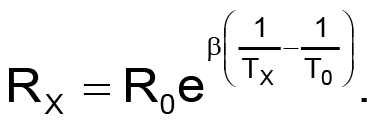
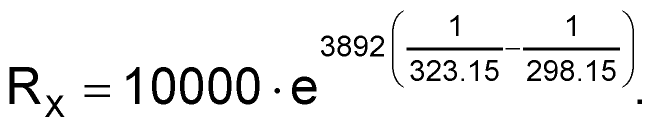
RX = 3643 (closest standard 1% value = 3650)
Now, if we conveniently choose VREF = 5 V for both input to RX and to the reference input of the ADC (since this is a ratiometric measurement, the absolute value of VREF is relatively unimportant) we can set:
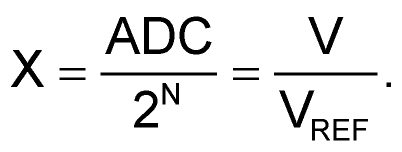
Then,

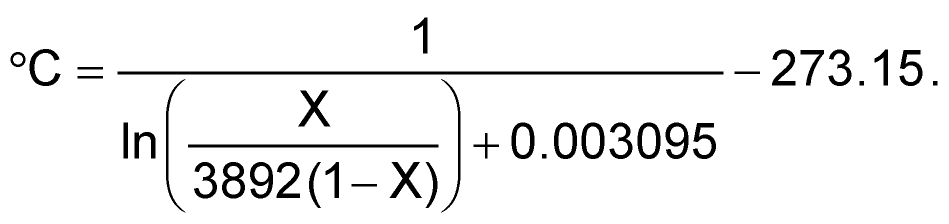
And the job is done!
Or is it? What about that dissipation (self-heating) factor (1.5 mW/°C)?
We obviously don’t want thermistor self-heating to significantly interfere with the temperature measurement. A reasonable limit for self-heating error might be half a degree and in the case of the 2152723803’s 1.5 mW/°C, this would dictate limiting maximum dissipation to no more than:
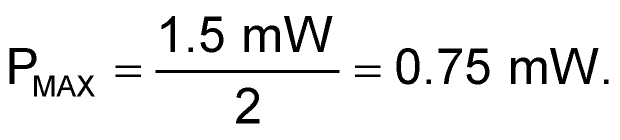
Dissipation maxes out to
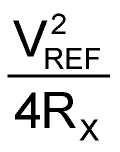
when RT = RX and in this case of VREF = 5 V will therefore be:
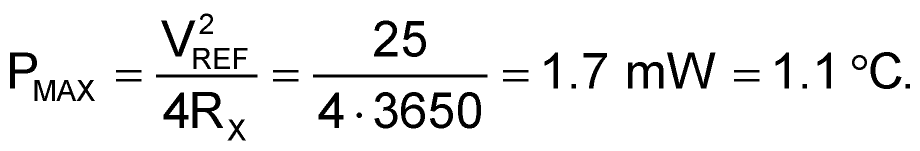
Yikes! That’s more than twice the stipulated maximum self-heating error. What to do? Not to worry, a solution is suggested by Figure 2.
 |
|
| Figure 2. | RVDD limits max thermistor self-heating to PMAX. |
Dipping again into the 2152723605 numbers and keeping VDD = 5 V:
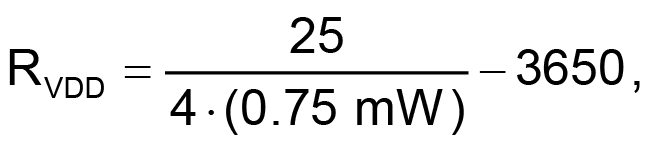
RVDD = 8333 – 3650 = 4.7k,
PMAX = 0.749 mW,
2.8 V < VREF < 5 V.
Note that if the Figure 2 math yields a zero or negative value for RVDD, then no RVDD is required, and the original Figure 1 circuit will work just fine.
Although VREF will vary with RT and therefore temperature, external-reference monolithic ADCs are typically very tolerant of VREF variations within the range shown and will perform accurate ratiometric conversions despite them.
And now the job is done! We just had to keep thinking positive.